Name: Colleen
Who is asking: Student
Level: Secondary
Question:
How is an ellipse like a circle?
In what way does an ellipse have a center?
How is a hyperbola similar and different to an ellipse?
How is a parabola similar a different to a circle ellipse and parabola?
Please help me with all or any of these questions.
Hi Colleen,| I really like this question. I have drawn a sketch of each of the conics that you mentioned. How are they the same and how are they different? The first fact that strikes me is that the hyperbola is in two pieces and each of the others is in one piece. The technical word is connected. The graphs of a circle, ellipse and parabola are connected but the graph of a hyperbols is disconnected. Imagine that these graphs are drawn on the floor. Stand on the graph of the circle and walk along the curve. Eventually you come back to where you started. The same property is true of the ellipse but it is not true of the parabola or hyperbola. The technical word here is closed. The circle and the ellipse have closed graphs but the ellipse and hyperbola do not. The center of a circle is special. If you stand at the center, face any direction and walk in a straight line, you eventually meet the circle. Also the distance you walk is the same, no matter which direction you chose. How is the center of an ellipse the same? How is it different. What other differences and similarities do you see? | 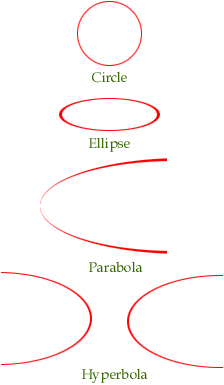 |
Penny