Quandaries and Queries
  

 

Person asking: Home schooling parent
Level: I don't know...High school I suppose

While teaching the multiplication table to my daughter I noticed an interesting pattern. It goes something like

...Take a whole number
...add two to it
...multiply the two numbers together
...add one to the resulting number
...it will now be equal to the original number plus one, squared. As an example 68=48...72=49 (6x8+1). but we noticed that this works with any number so it is [X(X+2)]+1=(X+1)(X+1) So my question is...Is there a name for this phenomenon? If so, what is the name? Certainly I'm not the first person to notice it.

 

 

Hi Melissa,

You can "picture" the equation [X(X+2)]+1=(X+1)(X+1) using the rows of pennies:

if [68]+1 pennies are arranged as a 6 by 8 rectangle and a single penny, as follows:


then you can move the last column to make it a row of 6 pennies on top:

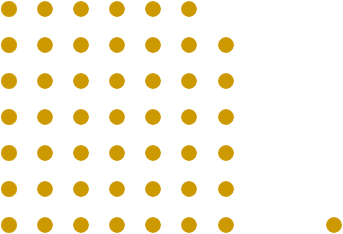
now you only need to put the single penny in the north-east corner to complete a 7 by 7 square of pennies.

Later on in school, your daughter will learn algebra so she will learn to expand expressions such as X(X+2), to get X2 + 2X, and also to expand (X+1)(X+1) to get X2 + 2X + 1 (using the identity (A+B)2 = A2 + 2AB + B2). Therefore she will have an algebraic justification of the identity [X(X+2)]+1=(X+1)(X+1):

[X(X+2)]+1 = [X2 + 2X] + 1 = X2 + 2X + 1 = (X+1)(X+1). Many students have difficulty with algebra. By showing your daughter these patterns in the multiplication table (rather than just treating it as data to memorize), you are helping her to overcome these difficulties which could arise later on.

Claude 		 
 

Go to Math Central