Dear Math Expert
I am a maths tutor. One of my year 12 students has given me this assignment. Many parts are ambiguous.
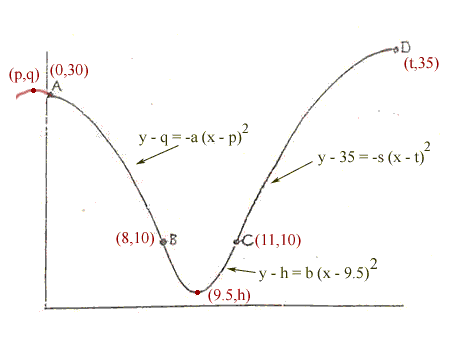
I am trying to determine the equation of the line segment AB. Given that A has an incline of 10 degrees below the horizontal, I am assuming that this parabola (although you can only see part of it) has been rotated 10 degree clockwise. Am I right in assuming this? If so, how do I derive the equation of the parabola as it will then not fit the general form y = ax squared +bx +c of a parabola.
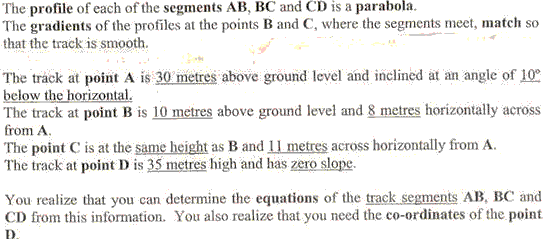
Also, how is one expected to find the x coordinate of D without the equation of this parabola. To find the equation you need the x-coordinate and therefore be able to find another point on the parabola in order to derive the equation using simultaneous equations.
I am just blown away. Please help.
Hi,
I labeled the diagram you sent with the given information, introducing variables where I needed them.
The main part of the text you sent is
In your question you asked "Given that A has an incline of 10 degrees below the horizontal, I am assuming that this parabola (although you can only see part of it) has been rotated 10 degree clockwise. Am I right in assuming this?" As you can see from the way I labeled the diagram, I assumed that the parabola that contains the segment AB has its vertex above and to the left of A at (p,q), and hence has equation
y - q = -a (x - p)2.
Since this parabola passes through A and B
30 - q = -a p2
and
10 - q = -a (8 - p)2
The slope of the tangent to this parabola at (0,30) is -tan(10o) so
2ap = -tan(10o)
These three equations are enough to determine a, p and q, and thus the equation of the parabola.
Can you complete the problem now?
Penny