Question: How do you find the area of a shaded sector of a circle?

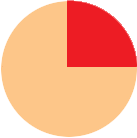
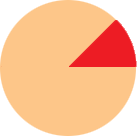
Natashia,

The area of the sector depends on the radius of the circle r and the measure of the angle t.

If the measure of the angle is 360^o the the sector is the entire circle and the area is r²

area = r²

If the measure of the angle is 180^o the the sector is one half the circle and the area is ¹/₂ r²

area = ¹/₂ r²

If the measure of the angle is 90^o the the sector is one quarter the circle and the area is ¹/₄ r²

area = ¹/₄ r²

If the measure of the angle is 45^o the the sector is one eighth the circle and the area is ¹/₈ r²

area = ¹/₈ r²

What you can see is that the area of the sector is a fraction of the area of the circle , and the fraction is the same fraction that the angle is of 360^o. Thus if the radius of the circle is r and the central angle of the sector has measure t then

the area of the sector is ^t/₃₆₀  r².

Penny