| |||
| Math Central | Quandaries & Queries |
|
Question from Dale, a student: Mathematically how can you calculate the distance of each side of a decagon? |
Dale,
Do you mean that the distance between a pair of parallel sides is 10 feet or the distance between two opposite vertices is 10 feet?
Harley
Dale wrote back
Yes, I mean that the distance between a pair of parallel sides is 10 feet .
Sorry for the confusion
Dale,
In the diagram below the length of AC is 5 feet, the angle ABC has measure 144/2 = 72 degrees and the angle CAB is a right angle.
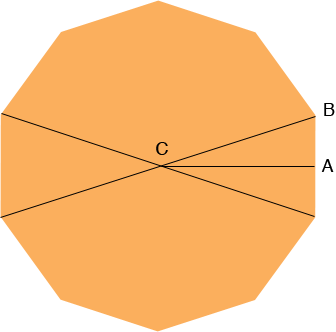
The tangent of the angle ABC is |CA| = 5 feet divided by |AB|, thus
tan(72o) = 5/|AB|
and thus
|AB| = 5/tan(72o) = 1.62 feet.
Hence the length of a side of the decagon is 2 × 1.62 = 3.24 feet.
Harley Weston
 |
||
Math Central is supported by the University of Regina and The Pacific Institute for the Mathematical Sciences.