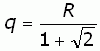Hi Griselda.

I'm glad you found the question from Avaline. It sounds like your question is better served with this diagram though:

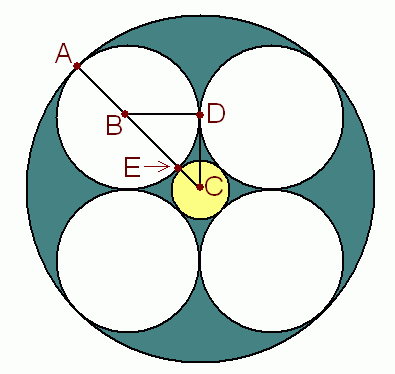
Let r = the length EC. That's what you want to find.

Let R = the length AC, that's what you are given: R = 10 cm .

Let q = the radius of the white circle.

So, AB = q, BD = q, BE = q, DC = q.

Since triangle CDB is a right triangle, you know the length of BC using Pythagorus:

But since AC = AB + BC = AC, we know

This means

However, we also know that BE = AC - AB - EC, so

This makes

So the two fractional expressions are both equal to q, which you don't really care much about. However, this means they equal each other. So set up the equation where they equal each other, solve for r, then plug in the value of R and find the radius of the small circle in the center.

Hope this helps,
Stephen La Rocque.