| |||
| Math Central | Quandaries & Queries |
|
Question from Robin, a student: A person is going to arrange a loan at 3 mill. he will pay 150000 back each year , so it take 20 yrs. to finish. In addition he pays 5% interest of what is leaft each year. How do I find the sum-function which shows the total sum he have payed? |
Hi Robin.
Let's write out the first few years to examine the pattern closely:
| End of year number | Balance before payment | Fixed Payment | Balance carried forward | 5% Interest on Balance carried | Cumulative Payment Total |
| 1 | 3 000 000 | 150 000 | 2 850 000 | 142 500 | 192 500 |
| 2 | 2 850 000 | 150 000 | 2 700 000 | 135 000 | 577 500 |
| 3 | 2 700 000 | 150 000 | 2 550 000 | 127 500 | 855 000 |
The cumulative payment total (CPT) is the previous CPT + the fixed payment + the interest for the current year.
The interest for year n is the balance carried forward times 5%. The balance carried forward for year n is $3 000 000 minus $150 000 times n.
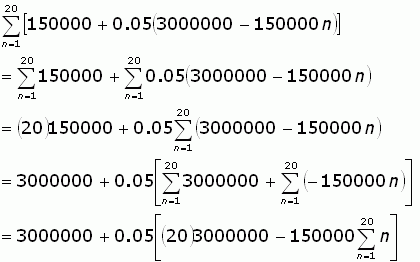
So CPT(n) = CPT(n-1) + 150 000 + 0.05 * (3 000 000 - 150 000n).
Another way of writing this is by using summation. Remember that a sum of a sum is a sum, so we can simplify the summation in steps:
Now the remaining summation is just the sum of the first few natural numbers. This is a well-known sum: the sum of the first A natural numbers is equal to A(A+1)/2, so we complete the problem as follows:
There's the result. A total of $4 425 000 would be paid out.
 |
||
Math Central is supported by the University of Regina and The Pacific Institute for the Mathematical Sciences.