| |||
| Math Central | Quandaries & Queries |
|
Question from John: I need to calculate the volume of a feed hopper, and I'm not sure how to break it down. The top of the hopper is 36" x 36", it is 30" deep, and ends at a 6" x 6" plate. One side of the hopper is straight top to bottom, of course tapering on two sides to meet at the plate. The other three sides angle down at about 75 degrees. I need to determine the cubic foot volume of this hopper (it is used for ground coffee) so I can configure a vibrator to knock down residual grounds. Thanks. |
John,
The taper you gave cannot be correct for all three other sides - it must be steeper on the two opposite sloping sides and less steep on the one facingthe vertical side. Fortunately, by Cavalieri's principle, we don't need to know the taper, just the cross section at each level. (Imagine a stack of horizontal cards which together had the shape of the hopper. Provided the cards stay the same size we can slide them around as we like and the total volume doesn't change.)
So it's the frustum of a pyramid of base 36" square, untruncated height 36", with a 6" square 6" high pyramid removed from the end.
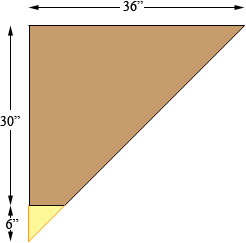
For a pyramid V = 1/3 Bh so the hopper volume is
1/3 (362 × 36) - 1/3 (62 × 6) = (363 - 63)/3 in3 = 15480 in3
-RD
 |
||
Math Central is supported by the University of Regina and The Pacific Institute for the Mathematical Sciences.