Hi Karen,

I drew a sketch of your tipi.

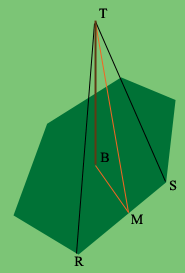
RS is one side of the base, M is the midpoint of RS, B is the base of the central pole and T is its tip. The length of TB is 5 feet and the length of BM is 3 feet. Triangle TBM is a right triangle so Pythagoras theorem gives is

|TM|² = |TB²| + |BM|² = 5² + 3² = 34

and hence

|TM| = 5.83 feet or 5 feet 10 inches.

Now turn your attention to the hexagonal base.

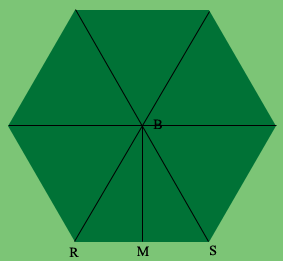
The measure of angle SBR is 360^o/6 = 60^o. Thus the measure of angle SBM is 30^o and the measure of angle MSB is 60^o. You know the length of BM is 3 feet and a little knowledge of trigonometry tells you that the length of MS is √3 feet. Hence the triangular fabric piece TRS has base length 2 √3 = 3.46 feet or 5 feet 6 inches and height MT 5 feet 10 inches.

If you want to know the length of the side TS you can use Pythagoras theorem again with triangle TMS to get

|TS|² = |MS|² + |TM²| = (√3)² + 32 = 41

and hence

|TS| = 6.40 feet or 6 feet 5 inches.

Good luck i this project,
Harley