Hi Phil.

Is the sketch below what you are making?

Let me cut away the solid sides and show you some of the useful internal triangles that will solve this problem:

We can solve your question by using the Pythagoras' Theorem (a² + b² = h²). It relates the lengths of the sides of a right triangle to the length of its hypotenuse.

First consider triangle ABC. We know the length of AC is the height (1100) and the length of BC is 225
( (800-350)/2 ). So AB is the hypotenuse and it is sqrt(1100² + 225²) which is about 1123 mm. That is the "slant height" of the large pieces.

Now look at triangle DEF. We know ED is 1100 and DF is 1325 ( (3000 - 350) / 2 ). That makes the slant height of the narrow pieces (EF) equal to sqrt(1325² + 1100²) which is about 1722 mm.

Finally, look at the triangle EFG. We know FG = 225 and (now) we know EF is 1722. So EG is sqrt(225² + 1722²) which is about 1737 mm.

Now we have all the measurements we need. Here are the two patterns we use for the four pieces:

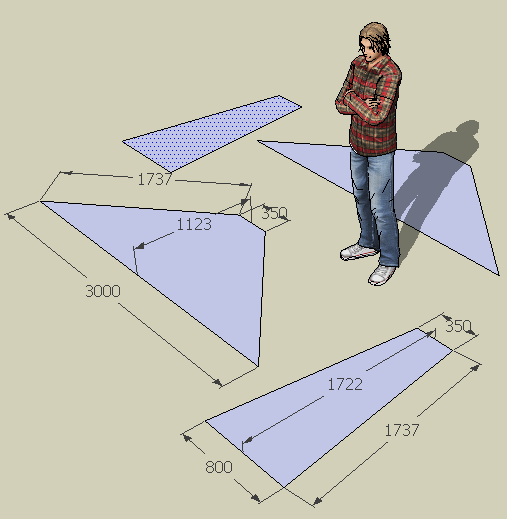
Hope this helps,
Stephen La Rocque.