Ronnie,

Is there more then one league night per week? Otherwise, there are some complications in doing it as you would like. Each team has to play 6 others in its division. Playing every other team in its division twice therefore takes at least 12 weeks. To do it in 12 weeks, every team would have to play on every league night. But there can only be three in-division games and one bye per division in each week. That means you can't accomplish it in 12 weeks.

A schedule might be possible if you go to 14 weeks where 6 teams play in-division and the seventh plays a crossover game. The remaining weeks may then not be needed, or can be interspersed as bye weeks.

Sorry for the discouraging news.
Victoria

Ronnie,

Victoria is correct but somewhat pessimistic. However, you need 19 weeks to realize your scheme, not the 18 you wished for. Here is an example of a 19-week schedule involving 14 teams in two 7-team divisions, where each team plays the other 6 in its division exactly twice, and plays each team in the other division exactly once. We divide the season into three rounds, the first two of which are 7 weeks long, while the final round lasts 5 weeks. For greater variety, you will probably prefer to play round 3 between rounds 1 and 2.

ROUND 1. Label the teams in each division from t1 to t7 and the weeks from w1 to w7. The pairings within the division are determined by the parallel diagonals of the regular 7-gon.

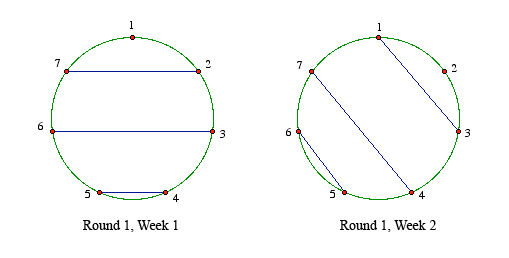
In week w you take the diagonals parallel to the tangent to the circumcircle at w. For example, as in the diagram for week 1, t2 plays t7, t3 plays t6, and t4 plays t5; furthermore, t1 of division A plays t1 of division B. The pattern continues for seven weeks: in week w team w-1 plays w+1, w-2 plays w+2, and w-3 plays w+3 (where if w-k is negative or zero, just add 7 to the number); furthermore team w of one division plays team w of the other in week w.

ROUND 2. Division A repeats its first round while Division B repeats the previous schedule shifted by a week. That is, Division B uses the pairing for the first round's week 2 during first week of the second round; in general, it uses the parings for the first round's w+1 during the second round's week w. As a result, during week w, team w of Division A plays team w+1 of Division B. For example, in week 1 of the second round, the first division repeats t2 plays t7, etc., while in Division B t1 plays t3, t4 plays t7, and t5 plays t6; furthermore, t1 from A plays t2 from B.

ROUND 3. This round lasts 5 weeks. In week w, w = 1 to 5, Division A's team t plays Division B's t+1+w. (Here you subtract 7 when the sum exceeds 7.)

Chris