| |||
| Math Central | Quandaries & Queries |
|
Alannah, a student: I have a word problem from my Calculus textbook that I can't figure out. Triangle ABC is inscribed in a semicircle with diameter BC=10cm. Find the value of angle B that produces the triangle of maximum area. I am supposed to set up an equation for the area of the triangle A=b x h/2 using Trig functions based on angle B to represent the base and height but I'm not sure how to do this when the side length given is not the hypotenuse. |
Hi Alannah,
Any triangle inscribed in a semi-circle, will have a right angle. If either angle B or C are 90o then the triangle would not be inscribed:

That means that angle A is 90o so BC must be the hypotenuse. We can use trig ratios to find length of the height and base of the triangle.
cosB = adjacent side to B/ hypotenuse = AB/10 ⇒ AB = 10 cos B
sinB = opposite side to B/ hypotenuse = AC/10 ⇒ AC = 10 sin B
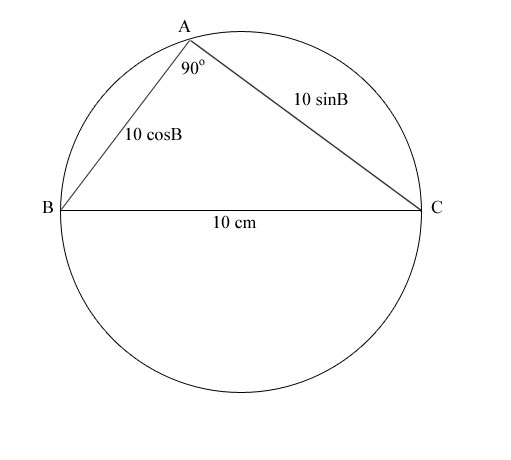
The formula for the area with respect to angle B
Area = 0.5bh=50 cosB sinB
Hope this helps,
Janice
 |
||
Math Central is supported by the University of Regina and The Pacific Institute for the Mathematical Sciences.