| |||
| Math Central | Quandaries & Queries |
|
Question from Mubashir, a student: A,B,C,D are the four corners of a rectangular plot marked on level ground. Given that the bearing of B from A is 040 degrees and that the bearing of C from A is 090 degrees. Calculate the bearing of |
Ho Mubashir,
I can help you with the diagram.
The bearing from $B$ to $ $A is the angle between the line segment $BA$ and north, measuring clockwise.
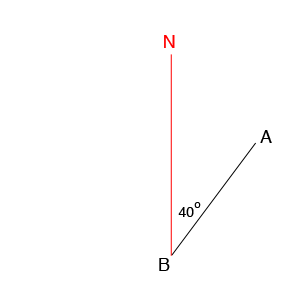
The bearing from $C$ to $A$ is 90 degrees and again the bearing is measured clockwise from north and thus $C$ must be on the east-west ray $L_1$ in the diagram below.
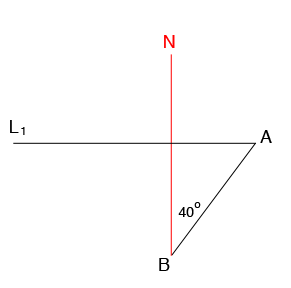
From the diagram the measure of the angle $BAC$ is 60 degrees and hence $AC$ is not a side of the rectangle. Hence the angle measure of the angle $CBA$ is 90 degrees. Draw a line through $B$ which is perpendicular to $BA$ and this line must intersect the line $L_1$ at $C.$
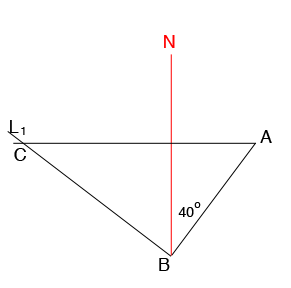
Finally draw a line through $C$ parallel to $BA$ and a line through $A$ parallel to $BC.$ These lines meet at $D$ the last corner of the rectangular plot.
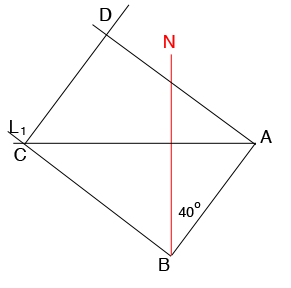
From the diagram you should now be able to determine the bearings required.
Penny
 |
||
Math Central is supported by the University of Regina and the Imperial Oil Foundation.