Hi Anagha,

I am going to draw the circle with its center at the origin $O$ in the Cartesian plane. I am going to identify a point $P$ on the circle by the measure $t$ of the angle between positive X-axis and the line segment from $P$ to $O,$ measured counterclockwise from the positive X-axis.

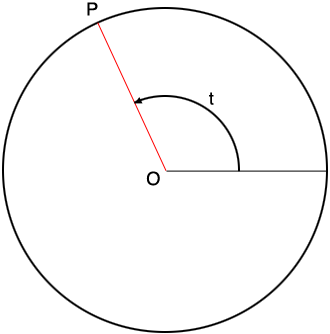
The problem is that $P$ is an unknown point on the circumference of the circle and there is a spinner attached at $O.$ Spin the spinner. What is the probability that it stops, pointing at $P?$

Suppose the probability is $Pr.$ Start by dividing the circumference of the circle with the X-axis. The two segments of the circumference are specified by

\[0^o \le t < 180^o \mbox{ and } 180^0 \le t < 360^{o}.\]

These segments are the same size and hence the probability that the spinner points at the segment containing $P$ is $\large \frac{1}{2}.$ Thus $Pr \le \large \frac{1}{2}.$

Further subdivide the circumference by adding the Y-axis to obtain the four segments

\[0^o \le t < 90^{o}, 90^o \le t < 180^{o}, 180^o \le t < 120^o\mbox{ and } 270^0 \le t < 360^{o}.\]

These segments are the same size and hence the probability that the spinner points at the segment containing $P$ is $\large \frac{1}{4} = \large \frac{1}{2^2}.$ Thus $Pr \le \large \frac{1}{2^2}.$

Further subdivide the circumference by adding the lines through the origin at $45^o$ and $135^{o}.$ This subdivides the circumference into $2^3 = 8$ segments and hence $Pr \le \large \frac{1}{2^3}.$

You can continue this process indefinitely, each time doubling the number of segments from the previous step. Hence at the $n^{th}$ step we have that $Pr \le \large \frac{1}{2^n}.$ We also know that the probability of any event is between 0 and 1 and hence, for all positive integers $n,$

\[ 0 \le Pr \le \frac{1}{2^n}.\]

As $n$ approaches infinity the sequence

\[\frac{1}{2}, \frac{1}{2^2}, \frac{1}{2^3}, \cdot \cdot \cdot, \frac{1}{2^n}, \cdot \cdot \cdot\]

approaches zero and hence we are forced to conclude that $Pr = 0.$

I hope this helps,

Harley