Carl sent this drawing

Hi Carl,

I am not sure how this goes together. Do you have a completed gate and if so can you send a photograph of the region I circled in your diagram? I assume there are bolts that go through the holes you drill but I am not sure where they go.

Harley

Carl replied

My apologies for delaying my response, but I am struggling with the way to explain this.

In the included drawing, Gate A is a regular square gate. The horizontal rails on top (C and D) have holes in them so that the vertical pickets (E) can go through them to the bottom rail (I). In Gate B the top rails (F and G) are curved with holes in them so that the pickets (H) can go though them as well. The problem is I cannot use the same hole pattern in rail G as in rail D because the arch changes the distance between the holes.

Is there a formula I can use to determine where to put the holes in a straight rail so that when I bend the rail the holes will line up with the bottom rail (J).

Carl

Hi Carl,

Your diagram didn't come through but I think I know now what you are asking. I assume the top curved rails are arcs of a circle. If you know the length of the chord AB in the diagram below and the height, |CD| of the highest point D above the chord then I can calculare the radius of the circle and work with that but there might be an easier approach.

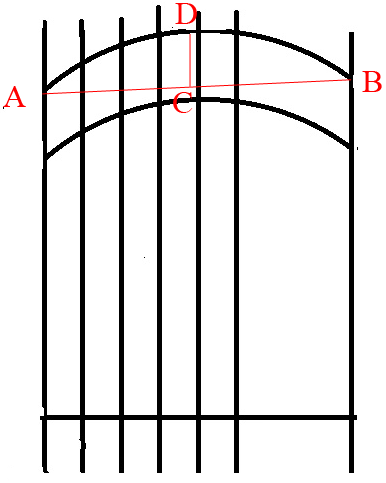
Suppose there are $n$ pickets counting the two at the edges of the gate, then there are $n - 1$ spaces between the pickets. Measure the length of the rail from $A$ to $B$ before you bend it. Call this length $L.$ Divide $L$ by $n - 1$ to find the distance between the holes. Drill $n - 2$ spaced $\large \frac{L}{n-1}$ units apart.

Do you think this will work?
Harley