Hi,

I can give you a construction argument. I'm not sure how intuitive it is but it has no arithmetic calculations.

First I traced the circular base of a 3.36 L paint can and cut it out.

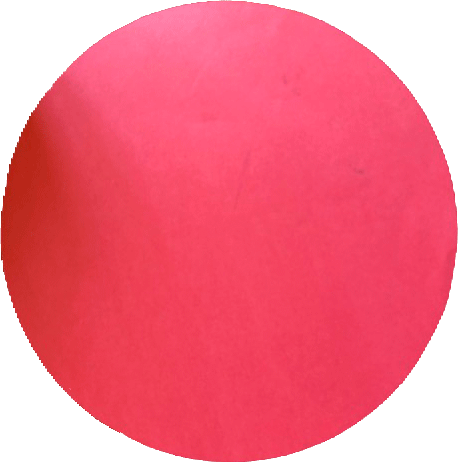
Next I wrapped a length of string around the can so that the string length was the length of the perimeter of the circle. I folded the string in half and then in half again to obtain the length of a side of a square with the same perimeter as the circle. I constructed a square with this side length and cut it out.

Finally I placed the square on top of the circle. Which has the larger area?

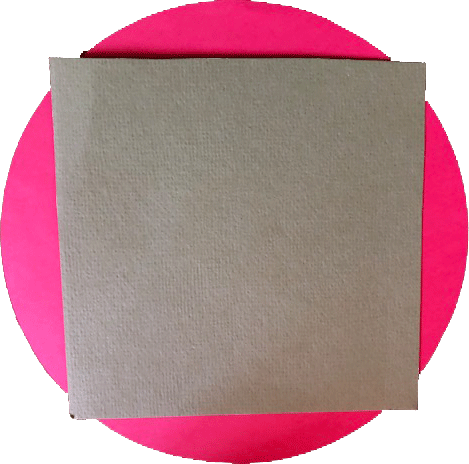
Try it. You need a reasonably large circle to see the difference,
Harley