Sender: MICHAEL
Subject: trig
Who is asking: Other
Level: Secondary
Question:
There is a cube box 3feet x 3feet x 3ft resting against a vertical wall on level ground.
Resting against the outside corner of the box is a ladder 10 feet tall,
this ladder is of course resting on the ground but also against the outside corner of the box and rests on the wall.
The question- the ladder is divided into two unequal section bounded by the box to the ground and the box to the wall. what are those dimensions?
Hi Michael
| In the diagram on the right O is the center of the ladder so |OA|=5. Let |PO|=t and |AB|=a. Since triangles ABP and PDE are similar and |DP|=3,
|
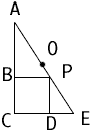
|
Penny
To return to the previous page use your browser's back button.