lim (1-cosx)/(x^2) as x-> 0

and

lim (tan3x)/x as x->0

Hi,

Many of the limits in first semester calculus that involve trig functions revolve around one fundamental fact. That fact is

The problem then usually involves some algebraic and/or trigonometric manipulation to use this fact. As is often the case in algebra and trigonometry you need to see a pattern somewhere.
   In your first problem the numerator is 1 - cos x and I know that

I also know that

and hence I can proceed as follows.

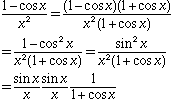
Thus

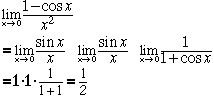
In your second problem tan3x = sin3x/cos3x and thus

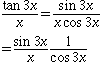
For the first factor to be of the form sint/t so that you can apply the fact mentioned above you need t = 3x so multiply the numerator and denominator by 3 to get

Now take the limit as x->0.

Cheers,
Harley