Who is asking: Student
Level: Middle
Question:
What is the difference between a regular shape and an irregular shape? Are a rectangle and a triangle regular or irregular shapes? Thank you
Hi Samuel,
"regular' has both a general meaning and a specific geometric meaning. In all cases, it concerns how much the various parts of the objects are 'the same'.
Consider the rectangle:
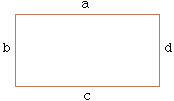
The sides a and c have the same length. The sides b and d have the same length. All the angles are the same. A sense of the the regularity comes from how you can pick it up turn it, and drop it back down and it still look the same. [These are called symmetries of the object.] Say pick it up and turn it 180 degrees: a goes to c, b goes to d. The property 'same length' now means something more: you can rigidly move it so those sides coincide. Say you pick it up and flip it over around a vertical line through the center (through ac).
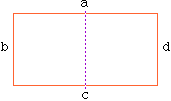
Side b goes to side d, sides a and d go on top of themselves. Another symmetry. A final reflection would involve turning through the line bd.

This takes side a to side c.
In fact, trying combinations of these, you can take any corner
of the rectangle onto any other corner. All the corners are
sort of 'the same'.
A square would have more symmetries than a rectrangle.
Any side could be taken to any other side, any corner to any other corner.
This has quarter turns as well as half-turns, mirrors through the corners
as well as the vertical and horizontal mirrors of the rectangle.
We would say that the square is MORE REGULAR than the rectangle,
because there are more symmetries. As a related fact, more parts
are the same.
For triangles:
- some have no two sides the same, so they are not regular.
- some have two sides the same. That also guarantees that
two of the angles are the same. These are isosceles.
Such triangles do have a mirror through the vertex (corner)
where the equal sides meet and the middle of the edge
opposite this vertex. That edge joins the two equal angles.
- some have all three sides the same. That goes with having all three angles the same. (Get one and the other follows.) For these 'equilateral triangles', there are lots of symmetries: taking any side to any side, any corner to any other corner. This would certainly be called regular.
When you go to larger shapes (five-sided or six-sided) you can look for more symmetries. If any side can be taken to any other side and any angle can be taken to any other angle, it is certainly called regular.
If some are the same and some are different, then I would not call it irregular. I might say it has some regularity or some symmetry.
There are lots of interesting questions to explore about regular shapes in space - like the cube or all the dice used in Dungeons and Dragons. Those dice are 'regular' at one level. Every face can be turned to be where any other face is. That is what makes if 'fair' when you roll it: all faces are 'the same' and have the same chance to turn up (or down). However, some of them do not have all corners or edges the same. So they are often called 'semi-regular' (not regular and not irregular).
Cheers,
Walter Whiteley