Surface area to volume ratio is very important determining factor in chemical reactivity. When materials have a high surface to volume ratio they react much quicker than when it is lower. For example: finely ground salt dissolves much quicker than coarse salt

An increased surface area to volume ratio also means increased exposure to the environment. The many thin tentacles of a jellyfish increase the surface area allowing more of the surrounding water to be sifted for food.

Greater surface area allows many organs to function more efficiently. The lung is an organ with numerous internal branches that increase the surface area through which oxygen is passed into and carbon dioxide is released from the blood. The intestine has a finely wrinkled internal surface, increasing the area through which nutrients are absorbed by the body. The outer layer of the brain, the cortex, is where most “thinking” occurs -- more surface area for the cortex, the more capacity for thinking. Brains are limited by skull size and mammal brains have many folds which increase the surface area in a fixed space. The surface area to volume ratio is increased allowing for more cortex and higher level processing.

Concept Properties Discovery Lessons

Activity 1
Each group should obtain at least 16 linking cubes. Divide the linking cubes into 2 groups of 8 linking cubes. Each shape will have a volume of 8 cubic units.

1. Make a shape with the minimum surface area.
2. Make a shape with the maximum surface area.
3. How do the shapes differ?
4. Most heat loss is through the skin so the ratio of skin surface area to volume of the body determines the speed with which heat is loss. Hypothermia is when a warm blooded animal loses too much heat. How would experience hypothermia quicker: an adult or a child? Why?

Activity 2
Compare Surface Area to Volume Ratio of Cubes and Spheres.

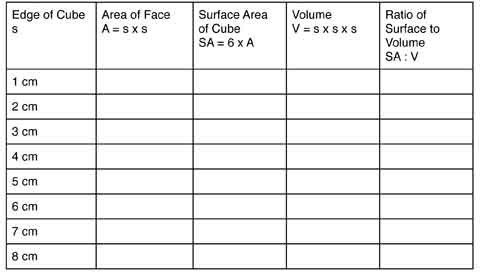
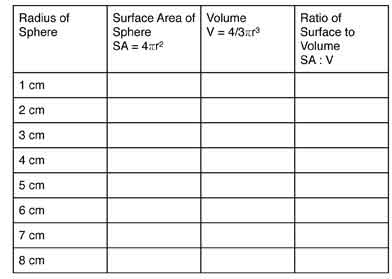
Answer the following questions:

1. For each shape, which size has the largest ratio of surface to volume?
2. For each shape, which size has the smallest ratio of surface to volume?
3. As the size increases, what happens to the ratio of surface to volume?
4. Considering each size, which shape has the largest ratio of surface to volume?
5. Most heat loss is through the skin so the ratio of skin surface area to volume of the body determines the speed with which heat is loss. Hypothermia is when a warm blooded animal loses too much heat. How would experience hypothermia quicker: an adult or a child? Why?

PDF - Activity 2

Activity 3
Set up a spreadsheet and calculate surface area, volume and volume/surface area for cubes, spheres, and tetrahedron for 1-20 cm. For each shape, determine volume divided by surface area.

Analysis

1. Create a line graph for Volume/surface area for each shape.
2. What type of graph is it?
3. Which shape has the largest volume to surface area ratio?
4. Which shape has the smallest volume to surface area ratio?
5. As the size increases, what happens to the ratio of volume to surface?

PDF- Activity 3

Suggested Follow Up Activities

Compare the melting rates of a large block of ice compared to many small ice cubes of
about the same total mass (spread out of course).

Compare the dissolving rates of a cube of sugar and loose sugar of the same amount total mass in warm water.

Make various 3D shapes out of sponges or foam. Cut each of the shapes in half and place floating in dyed water simultaneously. Observing the rate of absorption, which shape absorbs the dye the fastest?

Research Bergmann’s Rule that correlates latitude with body mass in animals.

Research Allen’s Rule that relates limb length with climate.

References:
"Surface area to volume ratio." Wikipedia, The Free Encyclopedia. 14 May 2008, 21:41 UTC. 18 Aug 2008

Hypothermia, Frostbite and Other Cold Injuries: Prevention, Survival, Rescue and Treatment. Gordon G. Giesbrecht, James A. Wilkerson, Andrea R. Gravatt. The Mountaineers Books, 2006

“ Building Shape - Surface Area to Volume Ratio”, CLEAR, 18 Aug 2008.

"Surface Area to Volume." Don Yee, Ryan Belscamper. Ask A Scientist, General Science Archive. 19 July 2005. 18 Aug 2008.

"Changing Surface Area and Volume with Changing Shape." Introduction to Nanoscale Science: Surface Area to Volume Ratio Module, NanoEd Resource Portal. 18 Aug 2008.

"Human Brain Folds: It's All in the Shape." Brain Health & Puzzles. 19 Aug 2008.