 |
Diamond Slopes and Speedy Whales
via… One Identity
by
Gregory V. Akulov, teacher,
Luther College High School, Regina, Canada
and
Oleksii V. Akulov, teacher,
High School #328,Kyiv, Ukraine
|
|
Diamond Slopes and Speedy Whales via… One Identity
One Identity.$^1$ Show that $\tan^{-1} x + \tan^{-1} y = 2 \tan^{-1} \Large{\frac{s}{p + \sqrt{s^2 + p^2}}},$
where $s = x + y, p = 1 - xy.$
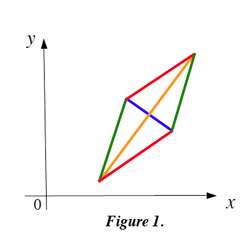 |
Diamond Slopes
If $m$ is the slope of rhombus diagonal drawn in the Cartesian plane (see Figure 1), and $l, n$ are the slopes of its sides, then
\[m = \frac{a}{b \pm \sqrt{a^2 + b^2}},\]
where $a = l + n, b = 1 - nl.$ Prove it.
Hint. You may use One Identity. |
 |
A Three-Speedy-Whales Problem.$^2$
auf Deutsch
en franšais
Once upon a time three whales were swimming along the north-south shoreline in the ocean near the island. Children at the island lighthouse observed whales’ motion and drew their position-time graphs in Cartesian coordinates. They noticed that intersections of three graphs form an isosceles triangle (see Figure 2). If the first whale swam at 7 m/s [N] and the second one at 1 m/s [N], what is the velocity of the third whale?
Hint. You may use Diamond Slopes Rule. |
Solve Equation: $2 - 2x - 2x^2 = x \sqrt{5 + 5 x^2}$.
Hint. Use One Identity to get $3 \tan^{-1} x = \tan^{-1} 2.$
______________________
$^1$ first appeared in 1999 volume 2 of Matematyka v Shkoli, the journal of the Ministry of Education and Science of Ukraine
$^2$ first appeared in 2010 volume 48:1 of delta-k, the journal of the Mathematic Council of the Alberta Teachers' Association
Copyright © March, 2010 by Gregory V. Akulov, Oleksii V. Akulov
|

