Hi Joe,

Sue answered a similar question from Angela, the daughter of a welder, a while ago but she wanted much more than the radius of the circle. You can use Sue's response to find the radius but if you only need the radius there is a more immediate method.

Below is a diagram of your cabinet with some labels and coordinate axes. For your cabinet q = 4 inches and p = 71/2 = 35.5 inches. The centre of the circle is somewhere further down on the y-axis.

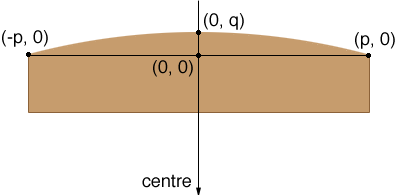
Suppose the centre of the circle is at (0, y₀) then the equation of the circle is

x² + (y - y₀)² = r²

where r is the radius of the circle.

Since the circle passes through (o, q) and (p, 0),

(q - y₀)² = r²
p² + y₀² = r²

Thus

(q - y₀)² = p² + y₀²

and simplification gives

y₀ = (q² - p²)/(2q)

From the diagram you can see that the radius of the circle is q plus the distance from the origin, (0, 0) to the centre of the circle, (0, y₀). Since y₀ is negative we drop the negative sign (using the absolute value function) and get

r = |y₀| + q

For your cabinet with q = 4 inches and p = 35.5 inches I got

y₀ = (q² - p²)/(2q) = (4² - 35.5²)/(2 4) = -155.5 inches

and

r = |y₀| + q = |-155.5| + 4 = 159.5 inches.

Penny