| |||
| Math Central | Quandaries & Queries |
|
Question from Larissa, a student: In a circle, the length of a chord AB is 4 cm and the length of the arc AB is 5 cm. Find the central angle theta, in radians, correct to four decimal places. Then give the answer to the nearest degree. I think I'm supposed to use Newton's method, but am not sure how to start with this problem. |
Hi Larissa,
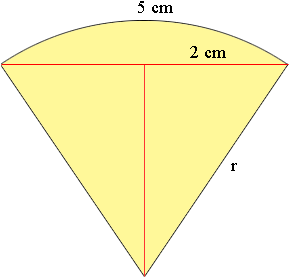
If the radius of the circle is r cm then you know that the length of the arc a is given by
a = r θ
Also from the diagram
sin(θ/2) = 2/r
Solve this equation for r and substitute into the first equation with a = 5. Now you can apply Newton's Method.
If you need more assistance write back,
Harley
 |
||
Math Central is supported by the University of Regina and The Pacific Institute for the Mathematical Sciences.